60.1K

Verified Solution

Link Copied!

We consider the following options strategy on ALPHABET and its total payoff at the expiry date of the short-term option.

Option | Type | Postion | Remaining Life | Strike

1 Call Short x 1 0 700

2 Call Long x 2 0.5 820

3 Call Short x 1 0 940

For the options with a remaining life, we use Black-Scholes-Merton (BSM) model to determine their values. We assume a volatility of 30% and a risk-free rate of 4%.

a) Compute the total payoff for S = 700, 820, and 940 (2 marks)

b) Use Excel to draw the payoff pattern for prices between $500 and $1100 with a step of $10. (3 marks)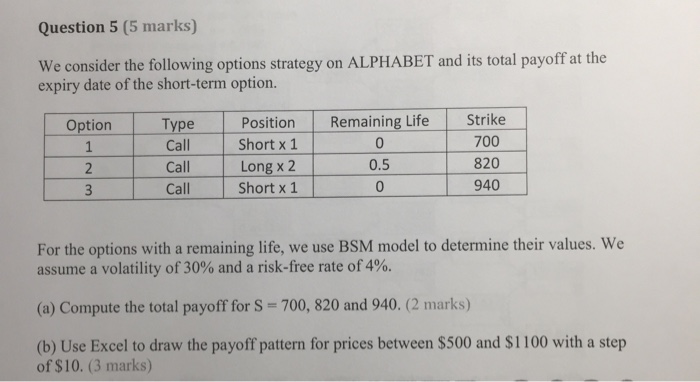

Question 5 (5 marks) We consider the following options strategy on ALPHABET and its total payoff at the expiry date of the short-term option. Option Remaining Life Type Call Call Call Position Short x1 Long x 2 Short x 1 Strike 700 820 940 .5 0 0 For the options with a remaining life, we use BSM model to determine their values. We assume a volatility of 30% and a risk-free rate of 4%. (a) Compute the total payoff for S = 700, 820 and 940. (2 marks) (b) Use Excel to draw the payoff pattern for prices between $500 and $1100 with a step of $10. (3 marks) Question 5 (5 marks) We consider the following options strategy on ALPHABET and its total payoff at the expiry date of the short-term option. Option Remaining Life Type Call Call Call Position Short x1 Long x 2 Short x 1 Strike 700 820 940 .5 0 0 For the options with a remaining life, we use BSM model to determine their values. We assume a volatility of 30% and a risk-free rate of 4%. (a) Compute the total payoff for S = 700, 820 and 940. (2 marks) (b) Use Excel to draw the payoff pattern for prices between $500 and $1100 with a step of $10

Answer & Explanation Solved by verified expert

See Answer