Logo
Home Study Material Contact Search
AI Chat FREE
  1. business
  2. accounting
  3. exercise 1. choose r\z and define z=ei. for each k

Exercise 1. Choose R\Z and define z=ei. For each kN we define Zk=1+z++zk. Show that...

80.2K

Verified Solution

Question

Accounting

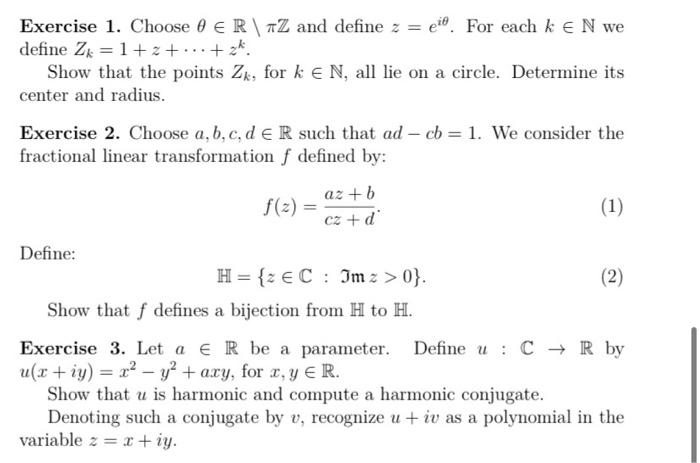image
Exercise 1. Choose R\Z and define z=ei. For each kN we define Zk=1+z++zk. Show that the points Zk, for kN, all lie on a circle. Determine its center and radius. Exercise 2. Choose a,b,c,dR such that adcb=1. We consider the fractional linear transformation f defined by: f(z)=cz+daz+b. Define: H={zC:Immz>0}. Show that f defines a bijection from H to H. Exercise 3. Let aR be a parameter. Define u:CR by u(x+iy)=x2y2+axy, for x,yR. Show that u is harmonic and compute a harmonic conjugate. Denoting such a conjugate by v, recognize u+iv as a polynomial in the variable z=x+iy

Answer & Explanation Solved by verified expert
Get Answers to Unlimited Questions

Join us to gain access to millions of questions and expert answers. Enjoy exclusive benefits tailored just for you!

Membership Benefits:
  • Unlimited Question Access with detailed Answers
  • Zin AI - 3 Million Words
  • 10 Dall-E 3 Images
  • 20 Plot Generations
  • Conversation with Dialogue Memory
  • No Ads, Ever!
  • Access to Our Best AI Platform: Flex AI - Your personal assistant for all your inquiries!
Become a Member

Other questions asked by students