Logo
Home Study Material Contact Search
AI Chat FREE
  1. business
  2. accounting
  3. consider a bond with a face value of $1,000 that

Consider a bond with a face value of $1,000 that sells for an initial...

80.2K

Verified Solution

Question

Accounting

Consider a bond with a face value of

$1,000

that sells for an initial price of

$700

. It will pay no coupons\ for the first nine years and will then pay

11%

coupons for the remaining 29 years. Choose an equation\ showing the relationship between the price of the bond, the coupon (in dollars), and the yield to maturity.\ A.

700=(110)/((1+i)^(9))+(110)/((1+i)^(9+1))+dots+(110)/((1+i)^(9+29-1))+(110)/((1+i)^(9+29))

\ B.

700=(110)/((1+i)^(9+1))+(110)/((1+i)^(9+2))+dots+(110)/((1+i)^(9+29-1))+(110)/((1+i)^(9+29))+(1,000)/((1+i)^(9+29))

\ C.

700=(1,000)/((1+i)^(29-9))

\ D.

700=(1,000)/((1+i)^(9+29))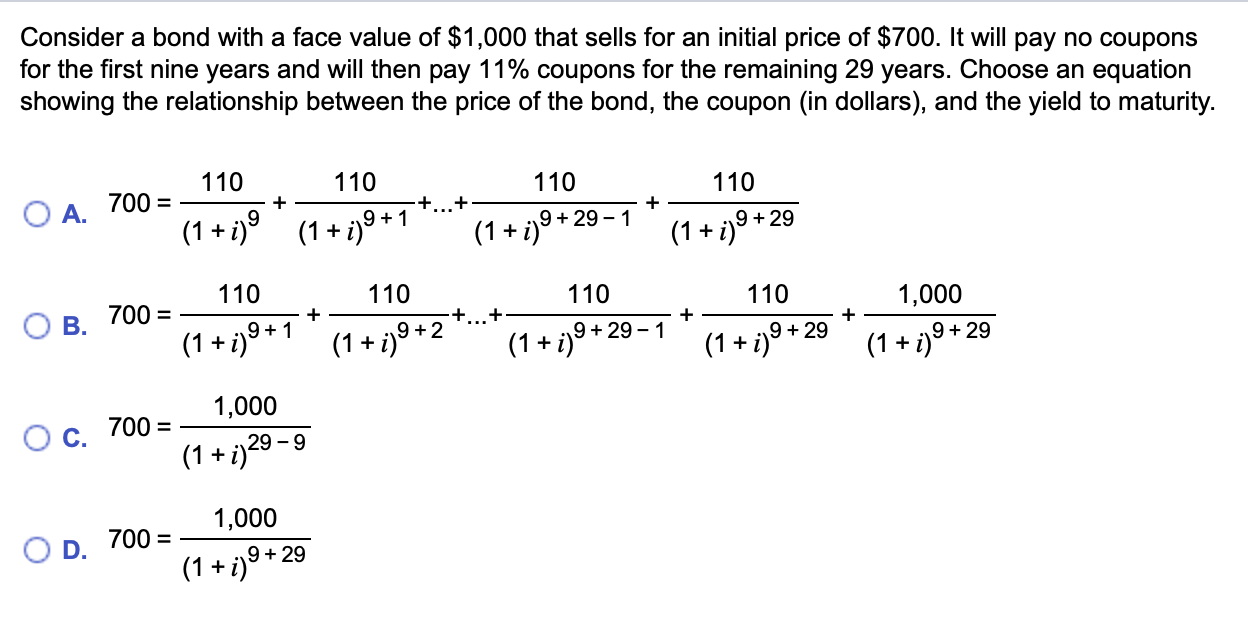
image

Answer & Explanation Solved by verified expert
Get Answers to Unlimited Questions

Join us to gain access to millions of questions and expert answers. Enjoy exclusive benefits tailored just for you!

Membership Benefits:
  • Unlimited Question Access with detailed Answers
  • Zin AI - 3 Million Words
  • 10 Dall-E 3 Images
  • 20 Plot Generations
  • Conversation with Dialogue Memory
  • No Ads, Ever!
  • Access to Our Best AI Platform: Flex AI - Your personal assistant for all your inquiries!
Become a Member

Other questions asked by students