70.2K

Verified Solution

Link Copied!

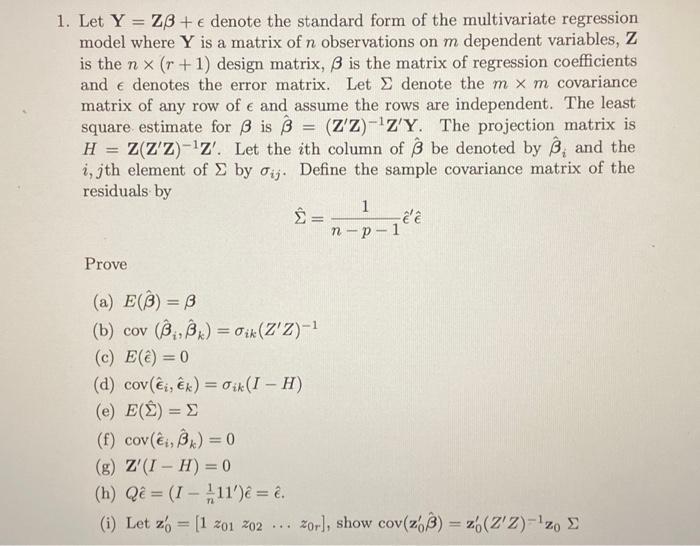1. Let Y=Z+ denote the standard form of the multivariate regression model where Y is a matrix of n observations on m dependent variables, Z is the n(r+1) design matrix, is the matrix of regression coefficients and denotes the error matrix. Let denote the mm covariance matrix of any row of and assume the rows are independent. The least square estimate for is ^=(ZZ)1ZY. The projection matrix is H=Z(ZZ)1Z. Let the i th column of ^ be denoted by ^i and the i,j th element of by ij. Define the sample covariance matrix of the residuals by ^=np11^^ Prove (a) E(^)= (b) cov(^i,^k)=ik(ZZ)1 (c) E(^)=0 (d) cov(^i,^k)=ik(IH) (e) E(^)= (f) cov(^i,^k)=0 (g) Z(IH)=0 (h) Q^=(In111)^=^. (i) Let z0=[1z01z02z0r], show cov(z0^)=z0(ZZ)1z0 1. Let Y=Z+ denote the standard form of the multivariate regression model where Y is a matrix of n observations on m dependent variables, Z is the n(r+1) design matrix, is the matrix of regression coefficients and denotes the error matrix. Let denote the mm covariance matrix of any row of and assume the rows are independent. The least square estimate for is ^=(ZZ)1ZY. The projection matrix is H=Z(ZZ)1Z. Let the i th column of ^ be denoted by ^i and the i,j th element of by ij. Define the sample covariance matrix of the residuals by ^=np11^^ Prove (a) E(^)= (b) cov(^i,^k)=ik(ZZ)1 (c) E(^)=0 (d) cov(^i,^k)=ik(IH) (e) E(^)= (f) cov(^i,^k)=0 (g) Z(IH)=0 (h) Q^=(In111)^=^. (i) Let z0=[1z01z02z0r], show cov(z0^)=z0(ZZ)1z0

Answer & Explanation Solved by verified expert

See Answer